Significance of Power Measurement in RF Devices

Introduction:

In the intricate world of Radio Frequency (RF) engineering, the measurement and expression of power play a pivotal role in ensuring the efficiency and reliability of devices. Understanding these power dynamics involves navigating the realms of decibels (dB) and logarithmic scales, where nuances can make a significant difference in RF system design. This exploration delves into the significance of power measurement, the relative nature of decibels, and the practical advantages of employing a logarithmic scale. Join us as we unravel the complexities of RF power expressions and discover how simplicity can be achieved through the artful use of dBm and dB.

Expression of Power

• Power measurement = most important measurement in RF devices.
• Power is energy transferred over time

Power measurement stands out as the pivotal metric in the realm of RF devices. Constant awareness of the output power level is imperative, whether you’re dealing with transmitters or power amplifiers. For RF engineers, mastering the nuances of power measurement is a career-long journey. At its core, power is the quantification of energy transferred over time and is conventionally measured in watts. Simplistically, one watt is the product of 1 Volt and 1 Ampere. However, when delving into the complexities of expressing power in a logarithmic scale, the unit dBm takes center stage. Notably, dBm uses 1mW as the reference point, offering a nuanced perspective on power levels.

Understanding power in RF devices extends beyond a mere measurement; it is a comprehensive exploration of energy dynamics over time. As RF engineers, the crux of our work lies in deciphering the transmitted power of a device. Whether it’s a transmitter or a power amplifier, the quest for accurate power levels is perennial. Power, measured in watts, is foundational—1 Watt equals 1 Volt multiplied by 1 Ampere. However, the complexity arises when we venture into expressing power in a logarithmic scale. The prevalent unit for this purpose is dBm, a logarithmic expression where 1mW serves as the reference point. This logarithmic approach allows for a more nuanced and practical assessment of power levels, offering insights critical for effective RF engineering.

Power is measured in watts

• 1 Watt = 1 Volt x 1 Ampere
• Power is usually expressed in:
• dBm which is a logarithmic expression using 1mW as reference

Measuring power is super important. It’s like the boss that decides how well the equipment works. RF engineers are always trying to figure out how much power is coming out. This whole thing is because power, which is like energy moving over time, is what keeps RF systems alive. We usually measure it in watts, which shows the basic connection between voltage and current. But, when we get into measuring power in a fancy way using a logarithmic scale, we turn to dBm. It’s like our special tool because it uses 1mW as a reference point. This way of measuring power becomes really handy in figuring out and understanding power levels in the busy world of RF devices.

Understanding Decibels in RF

In the intricate world of RF, power is often expressed in decibels (dB) or a logarithmic scale. This method, using dB, is a popular choice because it simplifies the comparison of two different power levels. When we express power in dBm, we are essentially comparing the power level we’re measuring with the reference of 1 milliwatt. The dBm is always relative to this 1mW reference, making it a powerful tool for simplicity in calculations.

In the realm of RF equipment and design, simplicity is key, especially when dealing with cascaded power gains and losses across different stages. Using decibels facilitates straightforward calculations of the overall system response. Unlike the traditional method of multiplication or division, working with decibels allows us to add or subtract gains or losses in terms of dB, providing a relative gain that streamlines the analysis of the entire system.

The formula for expressing power in dBm involves the logarithm base 10 of the power level being measured divided by the reference power (P_ref). The ‘m’ in dBm signifies the reference to 1 milliwatt and denotes an absolute power level, whether in watts or milliwatts. It’s crucial to remember that when we use dB, we are dealing with a ratio (P₁ to P₂), and the absence of ‘m’ implies that P₂ can be any type of power, making it a unitless, versatile measure of gain, loss, or isolation.

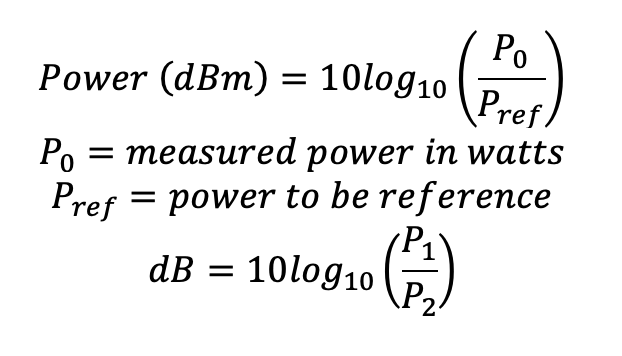
Understanding the difference between dBm and dB is paramount. While dBm is an absolute measure, dB represents a ratio between two power levels. So, if you see a value like 10dB, it doesn’t automatically mean 10dBm; the distinction lies in the reference and the nature of the measurement. This clarity ensures accurate communication and interpretation within the complex language of RF power expressions.

Example of Calculating Power:

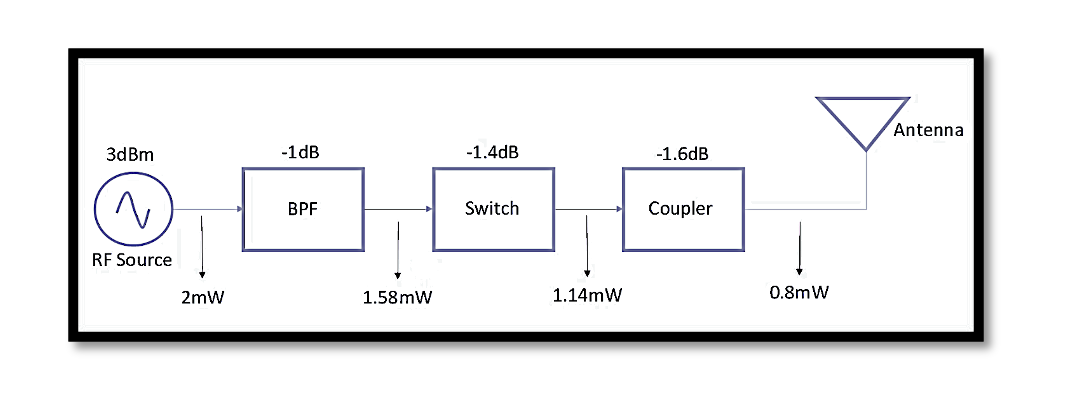
To illustrate the practicality of working with decibels in RF power calculations, consider a scenario where an RF source emits a 3dBm output, equivalent to 2 milliwatts. Your goal is to determine the power level just before your antenna, factoring in components like a bandpass filter, switch, and coupler.

The bandpass filter introduces a -1dB gain, effectively a 1dB loss. Importantly, this loss is relative, meaning it applies uniformly regardless of the input power—be it 3dBm, 10dBm, or 100dBm. The same principle holds for the switch and the coupler, emphasizing the relative nature of dB in contrast to the absolute dBm.

One key takeaway lies in the difference between employing milliwatts for calculations and utilizing a logarithmic scale system. If we were to compute each stage in milliwatts, accounting for the linear scale of gains and losses, the process involves multiplying factors, such as the -1dB gain of the bandpass filter. This multiplication continues through the switch and coupler, culminating in the final output power of 0.8 milliwatts.

However, embracing the logarithmic scale and expressing the power levels in decibels simplifies the entire process. Subtracting the 1dB loss of the bandpass filter, the -1.4dB loss of the switch, and the -1.6dB loss of the coupler from the initial 3dBm output becomes a straightforward task. The result is -1dBm, which, remarkably, matches the output power derived through linear calculations (0.8 milliwatts). This underscores the equivalence between dBm and milliwatts, showcasing the ease and efficiency brought about by the logarithmic scale in comparison to the linear scale of milliwatts.

Conclusion:

In the ever-evolving landscape of RF engineering, the importance of accurate power measurement cannot be overstated. The use of decibels and logarithmic scales emerges not just as a technical necessity but as a practical boon, simplifying intricate calculations in the face of cascading gains and losses. As we’ve witnessed, the relative nature of dB allows for a seamless comparison of power levels, while the absolute measurement in dBm provides a stable reference point. Whether considering gains, losses, or isolation, the marriage of dB and dBm presents a nuanced yet efficient approach to RF power calculations. In essence, this journey through the intricacies of RF power expressions showcases the elegance of simplicity achieved through the mastery of decibels.

Learn more about this topic by taking the complete course ‘Introduction to RF Testing Fundamentals and RF Test Architecture – RAHRF412’. Watch the course videos for more detailed understanding. Also checkout other courses on RF system and IC design on https://rahsoft.com/courses/. Rahsoft also provides a certificate on Radio Frequency. All the courses offer step by step approach.