Phase Noise Sources in PLL and transfer function reference phase noise

Phase-Locked Loops (PLLs) are fundamental components in various electronic systems, especially in communication and signal processing applications. PLLs are designed to lock the phase of the output signal to the phase of a reference signal, which is critical for maintaining signal integrity and synchronization. One of the significant performance metrics for PLLs is phase noise, which can severely impact the stability and clarity of the output signal. This blog delves into the sources of phase noise in PLLs and the derivation of the transfer function for reference phase noise, providing a comprehensive analysis based on the provided images.

Phase noise in PLLs originates from several sources, each contributing to the overall noise profile in different ways. The primary sources include:

1. Reference Oscillator Noise: This noise originates from the reference signal itself and can be a significant contributor, especially if the reference oscillator is not stable.
2. Phase Frequency Detector (PFD) and Charge Pump (CP) Noise: Noise generated within the PFD and CP circuitry due to inherent electronic imperfections and power supply variations.
3. Loop Filter Noise: The passive or active components in the loop filter can introduce noise, affecting the overall phase noise profile.
4. Voltage-Controlled Oscillator (VCO) Noise: The VCO is a critical component that converts the control voltage into a frequency. Its inherent noise characteristics can significantly impact the phase noise.
5. Feedback Divider Noise: Noise from the feedback divider, which scales the output frequency back to the reference frequency range, can also contribute to phase noise.

Reference Phase Noise and Transfer Function

Reference phase noise (ϕ_n,ref​) is one of the critical contributors to the overall phase noise at the PLL output. To understand how this noise propagates through the PLL and affects the output, we need to derive the transfer function. The images provided outline the steps to derive the transfer function and analyze its impact. Let’s break down the derivation process:

Deriving the Transfer Function

Basic PLL Setup: The PLL setup can be represented with a block diagram where the input phase noise is ϕ_in(t)=0, and the reference phase noise is ϕ_n,ref(t).

Closed-Loop Transfer Function: The closed-loop transfer function H_close(s) describes how the reference phase noise is filtered by the PLL:

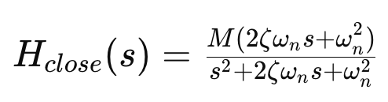
This function accounts for the damping factor (ζ) and the natural frequency (ω_n) of the PLL.

Output Phase Noise Calculation: To find the output phase noise (ϕ_out), we use the transfer function applied to the reference phase noise:

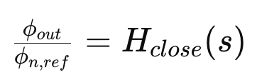
Substituting the transfer function into this relationship gives us:

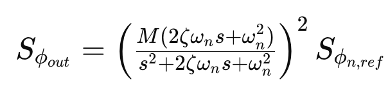
This equation shows how the reference phase noise is shaped by the PLL’s transfer function.

Phase Noise Spectrum:

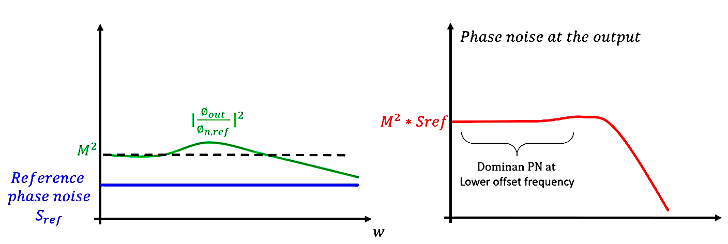
The phase noise spectrum at the output can be visualized as follows:

This plot indicates that the PLL acts as a low-pass filter for the reference phase noise. The magnitude response demonstrates that noise is attenuated at higher frequencies, showcasing the effectiveness of the PLL in suppressing high-frequency noise from the reference.

Analyzing the Transfer Function

The transfer function reveals that the PLL behaves as a low-pass filter for the reference phase noise. The magnitude response shows that the noise is attenuated at higher frequencies, leading to the conclusion that the PLL effectively suppresses high-frequency noise from the reference oscillator.

Phase Noise at Different Frequencies

1. Low Offset Frequencies: At lower offset frequencies, the phase noise is primarily influenced by the reference noise, as indicated by the flat response in the magnitude plot.
2. Higher Offset Frequencies: As the frequency increases, the phase noise contribution from the VCO becomes more significant. This is where the PLL’s filtering action is most effective.

The following image illustrates the phase noise behavior at different frequencies:

Trade-Off Between Reference and VCO Phase Noise Suppression

One of the critical considerations in PLL design is the trade-off between suppressing reference phase noise and VCO phase noise. The third image illustrates this trade-off clearly.

1. Low-Pass Filter (LPF) Response: Dominates at lower frequencies, effectively suppressing reference phase noise.
2. High-Pass Filter (HPF) Response: Dominates at higher frequencies, where the VCO phase noise becomes prominent.

The optimal design of a PLL aims to balance these responses to minimize the overall phase noise at the output. The intersection of the LPF and HPF responses indicates the balance point where the noise from the reference and the VCO are comparable.

Take our entry level course (Below) for free using coupon code RAHRF101BLOG

RF Fundamentals, Basic Concepts and Components – RAHRF101

For limited time take an additional 10% off of all our courses using coupon code RFCERT10

Rahsoft RF Certificate and courses 

Conclusion

Understanding the transfer function and phase noise sources in PLLs is crucial for designing systems with low phase noise performance. By analyzing the reference phase noise and its propagation through the PLL, engineers can optimize the PLL design to achieve the desired noise characteristics. The provided images and derived equations offer a comprehensive view of how reference phase noise is managed in a PLL, highlighting the importance of the transfer function in this context. This detailed analysis provides a solid foundation for anyone looking to understand or improve PLL performance concerning phase noise. By carefully balancing the trade-offs between different noise sources, designers can achieve optimal performance in their PLL circuits, ensuring stable and clear signal output for various applications

Learn more about this topic by taking the complete course ‘Phase Lock Loop System Design Theory and Principles RAHRF469’. Watch the course videos for more detailed understanding. Also checkout other courses on RF system and IC design on https://rahsoft.com/courses/. Rahsoft also provides a certificate on Radio Frequency. All the courses offer step by step approach.