Non-linearity and its effects in RF System

Linearity: Linearity is the property of a mathematical relationship or function, which can be graphically represented as a straight line. Linearity can be graphically represented as a straight line. We always expect to have this kind of outputs in our systems but actually in the real world it is bit hard and challenging to have a completely linear system, we end up having non-linearity.

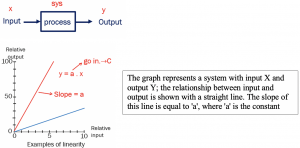
For example we have a system and the process here is amplification. The input to the amplifier is ‘x’ and output is ‘y’, we can graphically represent the relationship for x & y. For linear amplifier the relationship between x & y can be shown with a straight line and the slope of this line is equal to ‘a’. Where ‘a’ is the coefficient or gain and it is constant. So the output y=a.x.

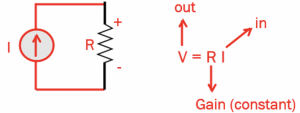
Another example of this system can be a resistor. In this circuit with input current ‘I’ and measuring the output voltage V across resistor ‘R,’ we will get V=R.I, where V is output, I is the input, and R is the constant gain. This resistor represents a linear system, and it has a linear behavior. output Y=a.X. However, it’s not quite true in real world because this resistance may change with temperature and due to temperature variations, this system will become non-linear. The two terms a₂ and a₃ in the below equation causes non-linearity.

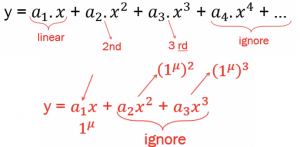
Non-linearity: In a non-linear system, as shown in equation (1), in addition to the first term we had in linear, there are two more terms called 2^nd order non-linearity and 3^rd order non-linearity. However, it is not limited to only two terms, and it can have more. As we proceed further, the terms after 3^rd order become small and low for comparison; hence, they can be ignored sometimes. When the system is really sensitive, these terms are also taken into account.

In cases where the value of ‘x’ is very small, the 2^nd order and 3^rd order terms are also ignored. Hence the system for a very small value of ‘x’ is linear. If a system shows non-linear behavior, it means ‘x’ holds considerable value and is not very small.

Example 1:

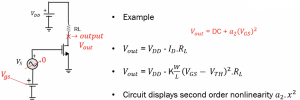
In this non-linear system example circuit, the input is V_gs, and the output is V_out, which is equal to V_OUT= V_DD-I_D.R_L. Read about the small signal analysis of the MOS transistor. The transistor in the circuit is working in saturation region with DC power considering AC is 0, then V_out can be further written as:

This circuit displays 2^nd order non-linearity (a₂.x²) and the relationship between input and output can be shown with a straight line. V_out shows a non-linear behavior in the equation: V_out=DC+a₂.(V_GS)².

Example 2:

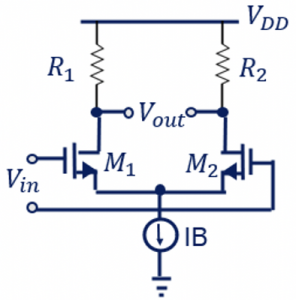
This example is of a MOS differential pair. The behaviour of this system is analysed using the given equations for differential pair. So, equation (3) shows the relationship between V_out and V_in in the given circuit.

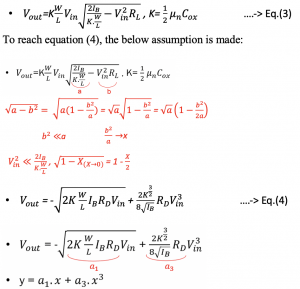
To reach equation (4), the few assumptions are made as shown. The condition is: b² is much smaller than ‘a’ and considered 0 and using the approximation we get equation (4). This circuit is now behaving like the 3^rd order non-linear system, and it doesn’t have a second-order term.

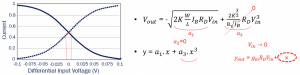
Making another assumption for this example circuit, the input V_in to the circuit is very small and is considered to be 0. This graph shows the curves for input voltage versus output current, and the intersection region V_in has a very small amplitude. Therefore, the term a₃can be ignored, considering it to be 0. Now we reached the point where the V_out behaves like a normal transistor with the value a1 only. Also, we read in previous sections that V_out = g_mR_DV_in.

When calculating the gain for our circuit in small-signal analysis, we always assume that our input has a low value like 1 micro volt for one stage for differential pair. But if you go and increase your value for V_in, the amplitude increases. In such a case, the system won’t show linear behavior. From this example and assumptions, we conclude that there are no 2nd order terms for differential systems; a₂ is always 0.

Learn more about this topic by taking the complete course ‘’RF Design Theory and Principles – RAHRF201’’.

Watch the course videos for more detailed understanding. Also checkout other courses on RF system and IC design on https://rahsoft.com/courses/

Rahsoft also provides a certificate on Radio Frequency. All the courses offer step by step approach.